| Problem | Solution |
|---|---|
 |
Symmetric with respect to the y-axis because:  |
 |
No symmetry with respect to either axis or the origin |
 |
Symmetric with respect to the x-axis because:
 |
 |
Symmetric with respect to the origin because:    |
 |
Symmetric with respect to the origin because:
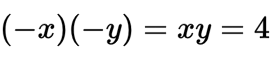 |
 |
Symmetric with respect to the origin because:
 |
 |
No symmetry with respect to either axis or the origin |
 |
Symmetric with respect to the origin because: |
 |
Symmetric with respect to the origin because:   |
 |

is symmetric with respect to the y-axis because
 |
 |

is symmetric with respect to the y-axis because
 |
 |

is symmetric with respect to the x-axis because
  |
Backdooring ATMs via Bootloader? These Hackers Showed It’s Still Possible
in 2025”
-
In a case that redefines the boundaries of modern cybercrime, a threat
actor known as UNC2891 has carried out a multi-vector cyber-heist targeting
ATM in...
2 weeks ago


0 comments:
Post a Comment